Sure! A geometric sequence is a sequence of numbers in which each term after the first is found by multiplying the previous term by a constant number. This constant number is called the common ratio.The general form of a geometric sequence is: a, ar, ar^2, ar^3, ..., where 'a' is the first term and 'r' is the common ratio.For example, if the first term of a geometric sequence is 2 and the common ratio is 3, then the sequence would be: 2, 6, 18, 54, ...To find any term in a geometric sequence, you can use the formula: a_n = a * r^(n-1), where a_n is the nth term, a is the first term, r is the common ratio, and n is the term number.Geometric sequences are used in many real-world applications, such as population growth, financial investments, and exponential decay in science.In summary, a geometric sequence is a sequence of numbers where each term is obtained by multiplying the preceding term by a constant ratio. This constant ratio is what distinguishes a geometric sequence from other types of sequences..
◂Math Worksheets and Study Guides Second Grade. Fractions Greater Than or Less Than 1/2
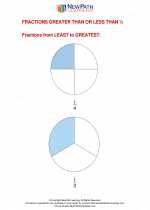
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Vocabulary/Answer key
Vocabulary/Answer key
