Straight Line
A straight line is a geometric figure that extends indefinitely in both directions. It is one of the fundamental concepts in geometry and is defined by two points through which it passes. The equation of a straight line can be expressed in various forms such as slope-intercept form, point-slope form, and standard form. Understanding the properties and equations of straight lines is crucial in various mathematical applications and fields of study.
Properties of a Straight Line
- Length: A straight line extends indefinitely and has no specific length.
- Slope: The slope of a line is a measure of its steepness and is defined as the ratio of the vertical change to the horizontal change between any two points on the line.
- Intercept: The point at which the line intersects the y-axis is known as the y-intercept, while the point at which it intersects the x-axis is called the x-intercept.
- Equation: The equation of a straight line can be expressed in different forms, such as the slope-intercept form (y = mx + b), point-slope form (y - y1 = m(x - x1)), and standard form (Ax + By = C).
Study Guide for Straight Line
When studying the topic of straight lines, it's important to focus on the following key areas:
- Understanding the concept of slope and how it relates to the steepness of a line.
- Learning how to find the slope of a line given two points or its equation in various forms.
- Mastering the different forms of the equation of a straight line and being able to convert between them.
- Practicing the application of straight lines in solving real-world problems, such as distance and speed calculations.
By grasping these fundamental concepts and practicing problem-solving, you can develop a strong understanding of straight lines and their applications in mathematics.
.◂Math Worksheets and Study Guides Fifth Grade. Ratio
Study Guide Ratio
Ratio  Activity Lesson
Activity Lesson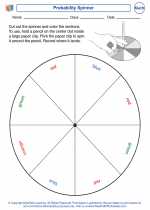 Probability Spinner
Probability Spinner  Activity Lesson
Activity Lesson Easy Money
Easy Money  Worksheet/Answer key
Worksheet/Answer key Ratio
Ratio  Worksheet/Answer key
Worksheet/Answer key Ratio
Ratio  Worksheet/Answer key
Worksheet/Answer key Ratio
Ratio  Worksheet/Answer key
Worksheet/Answer key Ratio
Ratio  Worksheet/Answer key
Worksheet/Answer key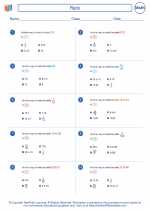 Ratio
Ratio  Worksheet/Answer key
Worksheet/Answer key Ratio
Ratio  Worksheet/Answer key
Worksheet/Answer key Ratio, Proportions and Percent
Ratio, Proportions and Percent  Worksheet/Answer key
Worksheet/Answer key Money Ratios
Money Ratios  Worksheet/Answer key
Worksheet/Answer key Animal Ratios
Animal Ratios  Vocabulary/Answer key
Vocabulary/Answer key Ratio
Ratio 

 Activity Lesson
Activity Lesson
 Activity Lesson
Activity Lesson
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Vocabulary/Answer key
Vocabulary/Answer key

The resources above cover the following skills:
Algebra (NCTM)
Use mathematical models to represent and understand quantitative relationships.
Model problem situations with objects and use representations such as graphs, tables, and equations to draw conclusions.