Secant in Geometry
A secant is a line that intersects a circle at two points. It extends beyond the circle and intersects the circle at two distinct points.
Key Terms:
- Secant: A line that intersects a circle at two points.
- Chord: A line segment whose endpoints lie on the circle.
- Tangent: A line that intersects the circle at exactly one point.
Properties of Secants:
When a secant intersects a circle, several properties can be observed:
- The line segment between the points of intersection is called a chord.
- Secant segments are outer parts of the secant line. They are formed by a secant and a tangent line that share an endpoint outside the circle.
- The product of the two segments of a secant line is equal to the product of the two segments of the other secant line.
Example:
Suppose we have a circle with center O and a secant line AB. Let's find the length of the segments created by the secant line.
 Circle with Secant Line AB">
Circle with Secant Line AB">In this example, we can calculate the length of the segments OA, OB, and AB using the properties of secants.
Practice Problems:
1. Given a circle with a radius of 5 units and a secant line that intersects the circle at points P and Q, calculate the length of the secant segment PQ if the distance between the circle and the secant line is 3 units.
2. In a circle with a radius of 8 units, if a secant line intersects the circle at points R and S, and the length of the secant segment RS is 12 units, calculate the distance between the circle and the secant line.
Conclusion:
Understanding the properties of secants and their relationship with circles is essential in geometry. Practice problems and visual examples can help solidify the concept of secants and their applications in various geometric scenarios.
.◂Math Worksheets and Study Guides Fourth Grade. Perimeter

 Activity Lesson
Activity Lesson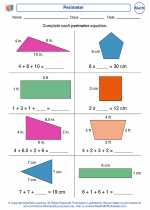
 Activity Lesson
Activity Lesson
 Activity Lesson
Activity Lesson
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Worksheet/Answer key
Worksheet/Answer key
 Vocabulary/Answer key
Vocabulary/Answer key
